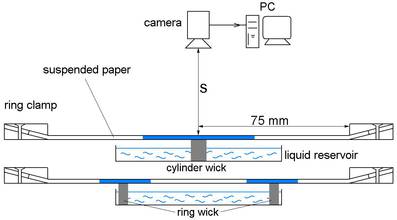
We have performed a theoretical and experimental study on the more basic situation when liquid spreads within a porous screen radially from an infinite reservoir. Figure 1 shows the arrangement. Our experiments are conducted with screen-like specimen of filter paper to ensure good observability and to avoid the displacement problem that would arise in a voluminous body. The paper screens are suspended on a ring clamp and from below a feeding wick touches the screen. The feeding wick rests in a reservoir containing the test liquid. As soon as contact between paper screen and feeding wick is established, the radial wicking process begins.

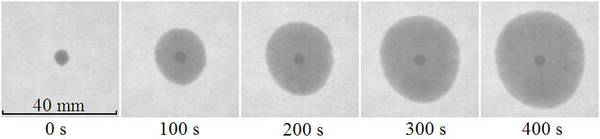
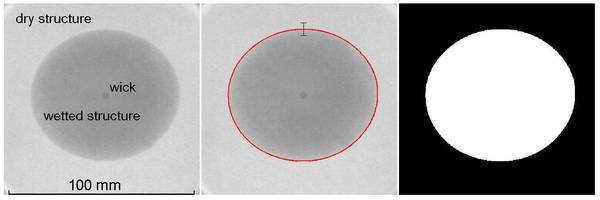
Figure 2 illustrates how a wetted spot grows in time for outward wicking. Inward wicking is achieved, by using a ring wick, for further information on that please look in the quoted literature. In regular intervals, a snapshot of the wetted spot is recorded. Subsequently, the wetted spot images are evaluated using Matlab routines to detect the perimeter of the spots, see Figure 3.

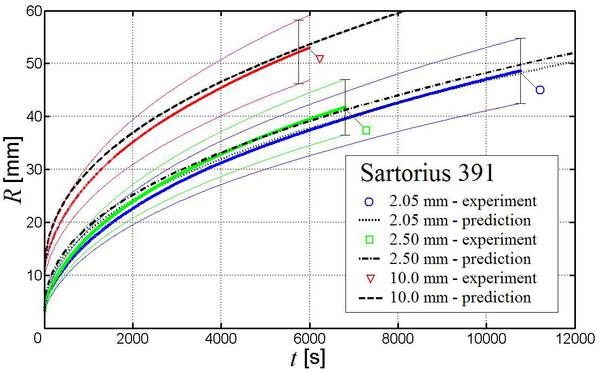
Our theoretical model predicts both outward and inward radial transport and is based on Darcy's law. While the model predicts the qualitative course of evolution for the spots, reference experiments are necessary for quantitative predictions. As example, Figure 4 shows the experimental results along with the prediction for one filter paper in which the test liquid spread from three different feeding wicks. As can be seen in the Figure, the results follow well the prediction within the expected error margins.